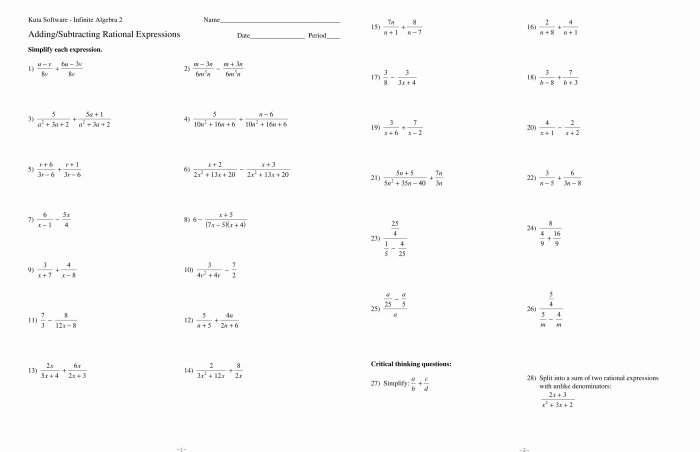
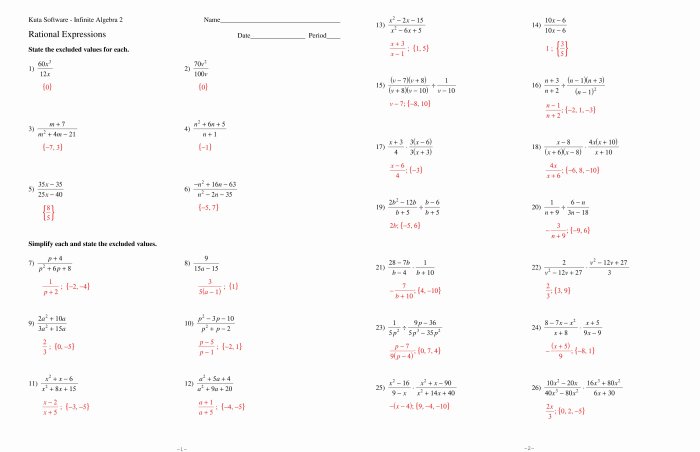
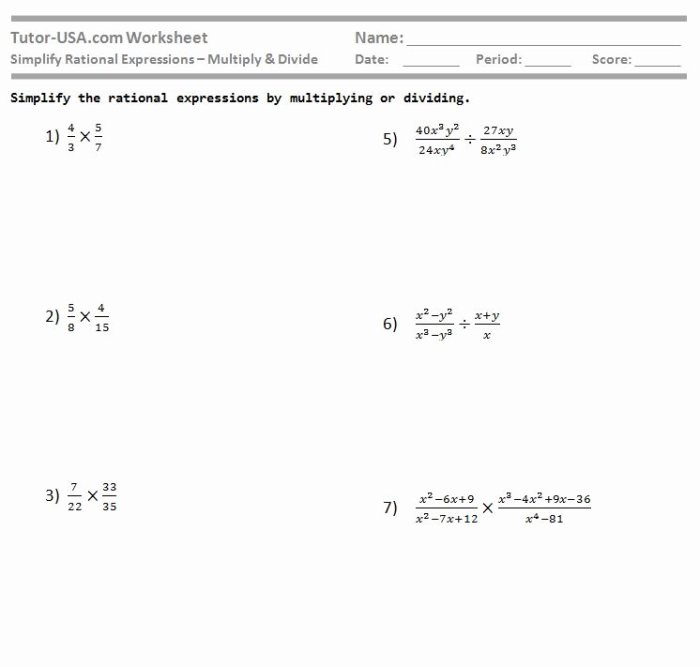
Embark on a journey of mathematical exploration with our comprehensive Simplifying Rational Expressions Worksheet with Answers. This valuable resource empowers you to master the art of simplifying rational expressions, providing a step-by-step guide and detailed solutions to enhance your understanding.
Delve into the intricacies of rational expressions, unraveling the methods of factoring, common factor division, and Least Common Multiple (LCM) to transform complex expressions into their simplest forms. Navigate the challenges of simplifying complex rational expressions with confidence, employing partial fraction decomposition and a structured approach.
Simplifying Rational Expressions Overview: Simplifying Rational Expressions Worksheet With Answers
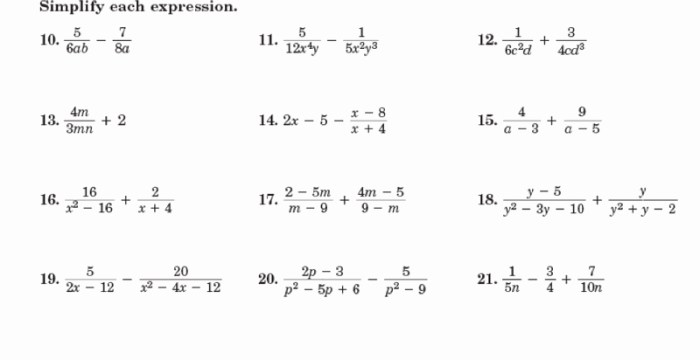
Rational expressions represent the quotient of two polynomials. Simplifying rational expressions involves reducing them to their lowest terms by eliminating any common factors in the numerator and denominator.
The steps involved in simplifying rational expressions include:
- Factoring both the numerator and denominator.
- Dividing out any common factors.
- Reducing the resulting expression to its lowest terms.
For example, the rational expression (x^2 – 1) / (x – 1) can be simplified to x + 1 by factoring both the numerator and denominator and dividing out the common factor (x – 1).
Methods for Simplifying Rational Expressions
There are several methods for simplifying rational expressions:
- Factoring and dividing out common factors:This method involves factoring both the numerator and denominator and dividing out any common factors.
- Using the Least Common Multiple (LCM):This method involves finding the LCM of the denominators of the rational expressions and multiplying both the numerator and denominator by the LCM.
For example, the rational expression (x + 1) / (x – 2) can be simplified to 1 / (x – 2) using the factoring and dividing out common factors method. The rational expression (2x) / (x^2 – 4) can be simplified to 2 / (x + 2) using the LCM method.
Simplifying Complex Rational Expressions, Simplifying rational expressions worksheet with answers
Simplifying complex rational expressions, such as those involving nested fractions or quadratic expressions, can be challenging.
Strategies for dealing with complex rational expressions include:
- Using partial fraction decomposition:This method involves breaking down the rational expression into a sum of simpler rational expressions.
- Multiplying by the conjugate:This method involves multiplying the rational expression by the conjugate of its denominator, which can simplify the expression.
For example, the complex rational expression (x^2 + 1) / (x^3 – 1) can be simplified to (x + 1) / (x^2 + x + 1) using partial fraction decomposition. The complex rational expression (x – 1) / (x^2 + 2x + 1) can be simplified to 1 / (x + 1) using the multiplying by the conjugate method.
FAQ Overview
What is the significance of simplifying rational expressions?
Simplifying rational expressions allows for easier manipulation, comparison, and evaluation. It reduces complex expressions to their simplest forms, revealing their true mathematical nature and facilitating further operations.
How does the Least Common Multiple (LCM) aid in simplifying rational expressions?
The LCM provides a common denominator for fractions, enabling the combination of like terms and the simplification of complex rational expressions. It ensures that the resulting expression is in its lowest terms.
What strategies can be employed to simplify complex rational expressions?
Partial fraction decomposition is a powerful technique for simplifying complex rational expressions. It involves breaking down the expression into a sum of simpler fractions, each with a linear or quadratic denominator.