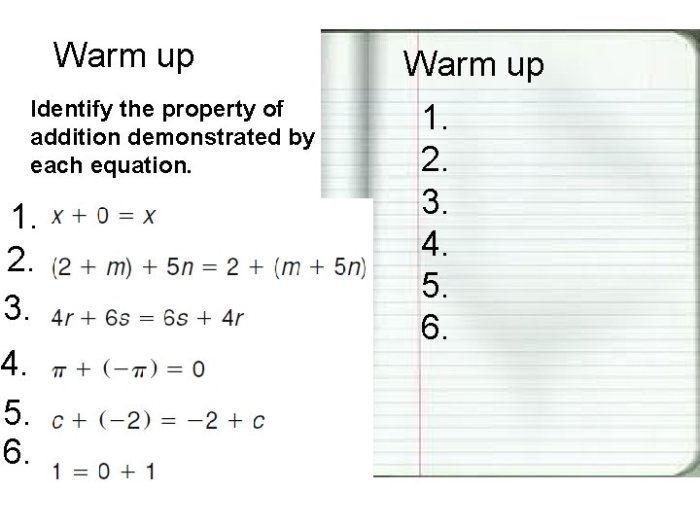
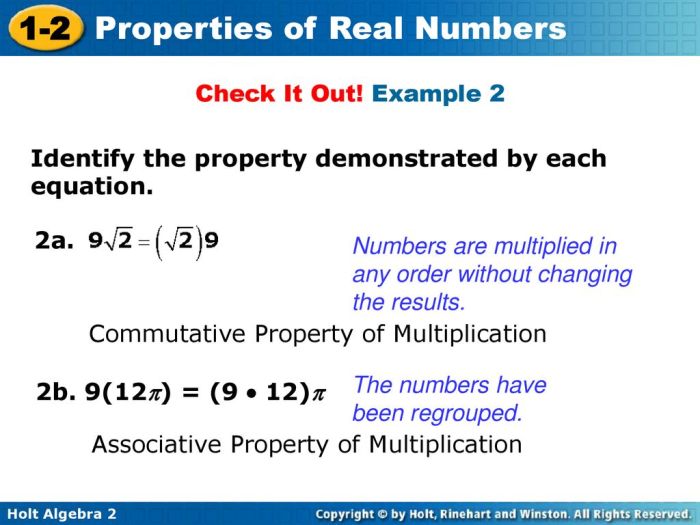
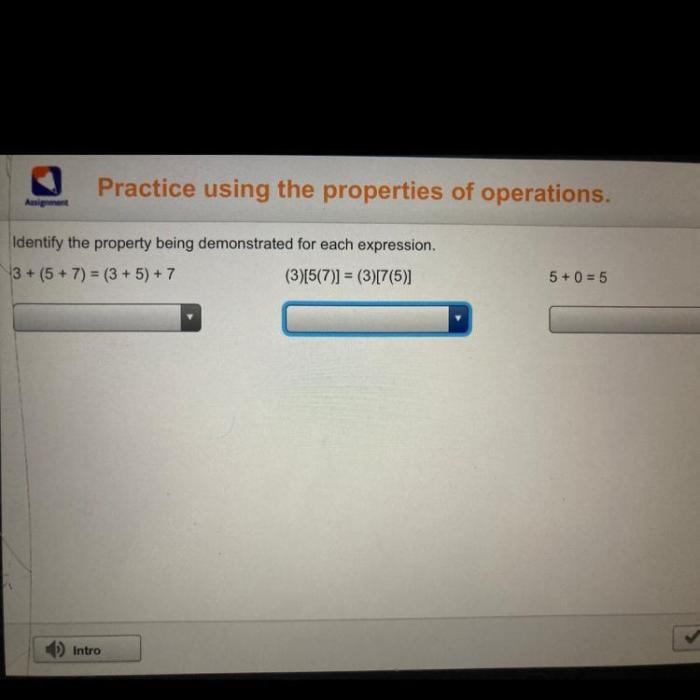
Identify the property demonstrated by the equation – In the realm of mathematics, equations serve as powerful tools for representing and analyzing relationships between variables. One key aspect of understanding equations lies in identifying the properties they demonstrate. By recognizing these properties, we unlock insights into the behavior and characteristics of equations, enabling us to solve and manipulate them effectively.
This exploration delves into the concept of equation properties, examining the role of mathematical operations, special properties, algebraic properties, and geometric properties in shaping the behavior of equations. We will uncover how these properties can be utilized to simplify, analyze, and solve equations, highlighting their practical applications in various fields.
Property Identification:

In mathematics, a property is a characteristic or behavior exhibited by an equation. Equations can demonstrate various properties, such as commutativity, associativity, distributivity, linearity, symmetry, and invertibility. Identifying these properties is crucial for understanding equation behavior, solving problems, and analyzing mathematical concepts.
Mathematical Operations and Properties:
Mathematical operations, such as addition, subtraction, multiplication, and division, play a significant role in determining equation properties. Commutativity, associativity, and distributivity are properties that govern how operations can be rearranged or grouped without altering the equation’s value. These properties facilitate algebraic manipulations and simplify equation solving.
Special Properties of Equations:, Identify the property demonstrated by the equation
Certain equations possess unique properties beyond the basic mathematical operations. Linearity refers to equations that can be expressed as a straight line, while symmetry describes equations that remain unchanged when variables are interchanged. Invertibility indicates that an equation can be reversed to find the original variables.
These properties provide valuable insights into equation behavior and enable efficient problem-solving.
Algebraic Properties and Equations:
Algebraic properties, such as the zero property, identity property, and inverse property, are closely related to equation properties. The zero property states that any number multiplied by zero equals zero, while the identity property asserts that any number multiplied by one equals itself.
These properties are fundamental in simplifying equations and finding solutions.
Geometric Properties and Equations:
Equations can also represent geometric shapes and properties. The slope of a line can be determined from its equation, and intercepts indicate where the line crosses the coordinate axes. Equations can be used to analyze geometric figures, such as circles, ellipses, and parabolas, providing valuable information about their size, shape, and position.
Applications of Property Identification:
Identifying equation properties has practical applications in various fields. In problem-solving, property identification can simplify equations, reveal patterns, and lead to efficient solutions. In modeling, equations with specific properties can represent real-world phenomena, enabling predictions and analysis. In data analysis, property identification helps identify trends, patterns, and relationships within data sets.
Questions Often Asked: Identify The Property Demonstrated By The Equation
What is the purpose of identifying equation properties?
Identifying equation properties helps us understand the behavior and characteristics of equations, enabling us to solve and manipulate them effectively.
How do mathematical operations affect equation properties?
Mathematical operations like addition and subtraction play a crucial role in determining equation properties, such as commutativity, associativity, and distributivity.
What are some examples of special properties of equations?
Special properties of equations include linearity, symmetry, and invertibility, which can be used to solve and analyze equations efficiently.
How are algebraic properties related to equation properties?
Algebraic properties like the zero property and identity property can be used to simplify and manipulate equations, making them easier to solve.
What are the practical applications of identifying equation properties?
Identifying equation properties has practical applications in various fields, including problem-solving, modeling, and data analysis, where it helps us understand and analyze complex systems.